
In Exercises 1–25, compute the inverse Laplace transform of the given function. Use the Convolution Theorem to find the Laplace transform of Using property (b) of Problem 15, find 1 ⁎ 1 ⁎ 1. ( f ⁎ g ) ⁎ h = f ⁎ ( g ⁎ h ) c.į ⁎ ( g + h ) = f ⁎ g + f ⁎ h d.į ⁎ 0 = 0, but f ⁎ 1 ≠ f and f ⁎ f ≠ f 2 in general. The Inverse Laplace Transform is one of the useful equations for turning a Laplace transform into a differential equation. Prove the following properties of the convolution of functions: This differential equations video explains some basic inverse Laplace transform examples using a Laplace transform chart. Due to the high level of complexity, tables of Laplace. For this we can resort to the use of the formula, but in most cases its use requires the execution of cumbersome and complex calculations. 20-28 INVERSE LAPLACE TRANSFORM Find the inverse transform, indicating the method used and showing the details: 7.5 20. 14.įind the convolution f ⁎ g of each of the following pairs of functions:į ( t ) = t, g ( t ) = e − t for t ≥ 0 c.į ( t ) = t 2, g ( t ) = ( t 2 + 1 ) for t ≥ 0 d.į ( t ) = e − a t, g ( t ) = e − b t ( a, b constants) e.į ( t ) = cos t, g ( t ) = cos t 15. When solving differential equations using the Laplace transform, we need to be able to compute the inverse Laplace transform. Use the result of part (a) and the derivative of the function F ( s ) = ln ( 2 + 3 s ), s > 0, to find its inverse Laplace transform. The inverse Laplace transform is known as the Bromwich integral, sometimes known as the Fourier-Mellin integral (see also the related Duhamels convolution. Show that the Laplace transform of t n f ( t ) is ( − 1 ) n F ( n ) ( s ), where F ( s ) = L. (This says that we can use the solution with any forcing function and zero initial conditions to compute solutions of other forcing functions.) 13.

Some of the exercises that follow will help you do this. In this case you have to apply the Laplace transform to the differential equation, solve for the transform L of the solution algebraically (via a solve command or by hand), use technology to find the inverse transform L − 1 ], and finally substitute the initial conditions.ĭetermine what your options are in using technology to solve IVPs via the Laplace transform. However, realize that the process of how it works is hidden, so you have to develop an understanding of what the system is really doing.īe aware that some computer algebra systems can find Laplace transforms and their inverses, but have no direct way of solving a linear IVP with these tools. If you have such an option at your command, learn to use it. In particular, some systems (for example, Maple) have sophisticated differential equation solvers with a “laplace” option for IVPs. Most computer algebra systems have built-in Laplace transform and inverse transform capabilities. Ricardo, in A Modern Introduction to Differential Equations (Third Edition), 2021 5.2.4 The Laplace transform and technology When we find our function of time, we will want to multiply by the Heaviside function to define it for t > 0.Henry J.
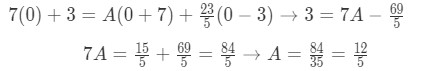
$ F(\lambda) => Inverse Laplace => f(t)H(t) $

That being said, having a working knowledge of partial fraction decomposition from calculus is necesarry to solve the Inverse Laplace Transform.Įssentially, now that we have a function in terms of lambda, we want to get it back to a function of t to obtain our solution. Unlike the Laplace Transform, the Inverse Laplace Transform does not have a long set of rules to memorize (hooray!). However, it can be shown that, if several functions have the same Laplace transform.
Inverse laplace transform table how to#
Great! We have made it halfway to where we want to be we know how to apply the Laplace transform, and now we need to learn how to apply the inverse Laplace Transform. Example 6.24 illustrates that inverse Laplace transforms are not unique.


 0 kommentar(er)
0 kommentar(er)
